Review of Linear Slopes

Economists use graphs (linear and non-linear) to represent ideal relationships between two economic variables. This is one way to do economic modeling. In economic modeling we are not reporting factual information (data points), we are summarizing what would be theoretically perfect  relationships.

Basics of Linear Graphs

All graphs illustrate the relationship between two variables, the independent variable and the dependent variable. The independent variable changes on its own or is changed by some other factor that is not reprented on the graph. The dependent variable changes in response to the change in the independent variable. You can think of the independent variable as "the cause" and the dependent variable as "the effect" or "the result". 

The simplest mathematical model we can create is a straight line. This is the simplest because it has only one cause, the independent variable, and one result, the dependent variable. It also has a constant slope, which means that the relationship between the two variables is not changing.

When the independent variable changes it is represented on the graph as sliding from one point on the line to another point on the same line. This is because the line maps out the value of the dependent variable for every change in the value of the independent variable.

The diagram below illustrates how to visually estimate the slope of the line without having to spend time actually measuring or calculating the numerical value of the slope.

The Slope of a Line

The slope of a line is measured by comparing the change in the vertical direction to the change in the horizontal direction between two points on the line. Mathematically, we calculate the slope by dividing the change along the vertical axis by the change along the horizontal axis ("the change in the rise over the change in the run").

However, at the introductory level we do not necessarily need to make these calculations to be able to interpret the meaning of a graph. We can visually estimate the value of the slope simply by identifying the sign (positive or negative) and the magnitude (size or steepness) of the slope.

Briefly, a line that is drawn from the bottom left to upper right has a positive slope. A line that is drwan from the top left to the bottom right has a negative slope. A flat line has a small slope, numerically close to zero. While a steep line has a large slope, numerically it is number that is far from zero, either very positive or very negative. These points are explained further below.
 

Positive versus Negative Slopes

The relationship between the price of a good and the amount that a consumer is able and willing to buy (i.e. the Demand Line) is a negative or inverse relationship, which is represented by a line (or convex curve) that is downward-sloping from upper left to lower right. It is a negative (or inverse) relationship because one of the variables is negative while the other is positive. They move in opposite directions. If price rises (grows positively), the quantity demanded by consumers falls (moves negatively).

In contrast, positive or direct relationships are represented as upward sloped lines, from bottom left to upper right. This is because both variables increase together OR decrease together. They move in the same direction. The Supply Line is the most common economic example of a positively sloped line. When the price of a good rises in the market producers are motivated to produce more of the good in order to make more profit per unit sold. So, both price and the amount produced (the quantity supplied) increase. Alternatively, if the price of the good falls, producers will cut production since they now make less profit per unit sold, or may even lose money at a lower price. In this case both price and the quantity produced fall or move in a negative direction together.


linearslopes


Flat versus Steep Slopes

A negative-sloped Demand line that is relatively flat indicates that consumers are very sensitive to small changes in Price and respond with relatively large changes in their purchasing behavior. Similarly, A postively sloped Supply line that is relatively flat indicates that Producers are very sensitive to small changes in Price because they respond with a bigger change in production.

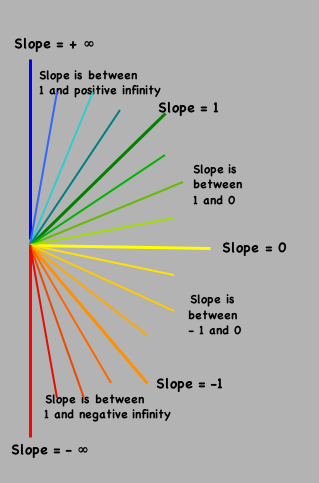
How do we know this?

Because a relatively flat Demand line indicates that a small change in the vertical variable (Price) corresponds to a larger change in the horizontal variable (Quantity). This is particularly clear when you compare a "flattish" Demand line to a steep Demand line, which shows that a large change in the vertical variable is associated with a small change in the horizontal variable. Of course, the same comparison holds when you compare a flat Supply line with a steep Supply line.

For example, compare the difference in the vertical to horizontal relationships of a slightly negatively sloped line (say a light yellow one) to a very steep negatively-sloped line (say the red-orange line). Also compare the difference in the numerical value of the slope of flat Supply line (light green) to a steep Supply line (dark blue).

 Being able to visually intepret both the mathematical concepts of graphs, as well as, the economic meaning of the graphs is a useful skill for introductory economics students.

This page is developed and managed solely by Philip R. Martinez.

Copyright 2003 - Philip R. Martinez and Lane Community College.

All Rights Reserved.